ON CONSTRUCTING 2D AND 3D EQUIDECOMPOSITIONS USING TESSELLATIONS AS A DISSECTION TOOL
 

F. AGUILÓ, M. A. FIOL, and M. L. FIOL



Name: Francesc Aguiló Gost, Mathematician, (b. Sa Pobla, Mallorca, Spain, 1962). 

Address: Dept Matemàtica Aplicada IV, Universitat Politècnica de Catalunya, Jordi Girona 1-3, Mòdul C3, 08034, Barcelona, Spain. 

E-mail: matfag@mat.upc.es

Fields of interest: Discrete Mathematics and Graph Theory.
 
 

Name: Miquel A. Fiol Mora, Telecommunication Engineer, (b. Palma, Mallorca, Spain, 1949). 

Address: Dept Matemàtica Aplicada IV, Universitat Politècnica de Catalunya, Jordi Girona 1-3, Mòdul C3, 08034, Barcelona, Spain. 

E-mail: fiol@mat.upc.es

Fields of interest: Integral Matrices, congruences in n-dimensional spaces, interconnection networks, connectivity of graphs and algebraic combinatorics.
 
 

Name: Maria L. Fiol Mora, Mathematician, (b. Palma, Mallorca, Spain, 1943). 

Address: Dept Didàctica de le Matemàtiques i Ciències Experimentals, Universitat Autònoma de Barcelona, 08193, Bellaterra, Barcelona, Spain. 

E-mail: ml.fiol@cc.uab.es

Fields of interest: Mathematics education, proportional reasoning and children’s geometrical thinking in the primary years.

Joint Publication: Periodic tilings as a dissection method, American Mathematical Monthly, Vol. 107 (2000), No. 4, pp. 341-352.
 

Abstract: We present a method to construct 2D equidecompositions of any two polygons with the same area. In the 3D case, we give equidecompositions of two parallelotopes with the same volume. Physical examples of 2D and 3D decompositions will be shown. These constructions are based on a general method to obtain equidecompositions of some n-dimensional polyhedra with the same volume, here referred to as equivalent tiles. This technique consists of a dissection of both tiles with a finite number of congruent pieces. The method is based on the superposition of some congruent (that is, with the same lattice) periodic tessellations obtained from the original polyhedra to be dissected. This superposition ‘cuts’ the first tessellation using the second one and so a finite number of the pieces are obtained. The finiteness of the number of these pieces is given by the periodicity of these cuts, because of the equivalence of the two involved tessellations. Let V={v1,...,vn} be a set of independent vectors in Rn, then the reticle generated by V is

Â={l1n1+l2n2+...+lnnn; l1, ...,lnÎ Z} 

We say that a tile TÎ Rn periodically tessellates the space if there is some reticle  such that the tilling T+ ={T+u;uÎÂ} is a partition of Rn (the borders of the tiles can overlap each other.) Two tessellations are congruents if they have linked to the same reticle. Then two tiles, T1 and T2 with the same volume, which periodically tessellate the space with congruent tessellations, can be equidecomposed.*
 

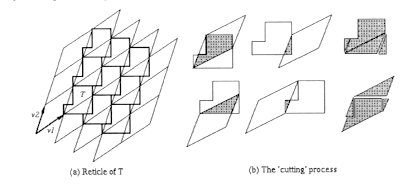
1. 2D EQUIDECOMPOSITIONS

Let us equidecompose some polygons in R2. Let V={v1,v2} and T as shown in Figure 1. Note that the borders overlap between them, a fact which is allowed. Note also that the rhombi defined by the tiling generated by V form a congruent tessellation with the one formed by T. Denote the tile T by T1 and the rhombus by T2. As T1 and T2 have the same area, they are equidecomposable.

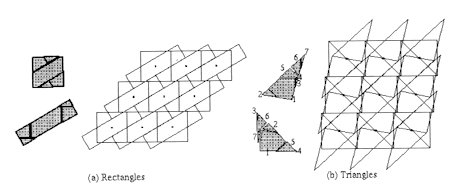
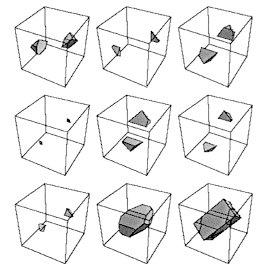
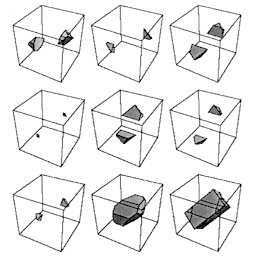
Figure 1. (a) T and its related reticle; (b) The ‘cutting’ process of the congruent pieces.

 

Let us dissect T1 and T2 in congruent pieces using these two tessellations. The process is depicted in Figure 1: fixing the T1 tessellation, we can cut the congruent pieces using the T2 tessellation, or vice versa (because of the congruence of the tessellations.) Note that the obtained four pieces can reconstruct T1 and T2, so giving an equidecomposition of these two tiles. This method also allows us to decompose any two parallelograms with the same area. Then we can equidecompose any two equivalent triangles (doubling them to two parallelograms.) Figure 2 shows two examples of these decompositions. Finally we can decompose any two polygonal regions of the same area by previously decomposing them into a number of equivalent triangles. 


Figure 2. Equidecompositions of rectangles and triangles.


 

2. 3D EQUIDECOMPOSITIONS

For two parallelotopes with the same volume in R3, we can also construct the related three dimensional tessellations. See Figure 3 for an example of a cube and a parallelepiped. The process to obtain the pieces of the decomposition is the same as in the two dimensional case. Figure 4 shows how the cuts look like for three pieces.


Figure 3. Two congruent 3D tessellations of cubes and parallelepipeds.


Figure 4. Obtention of three pieces of the decomposition. 

Finally we can see in Figure 5 and Figure 6 two maps on how to reconstruct the original parallelepipeds from the 15 pieces of the decomposition. Every pair of pieces in each map presents a central symmetry. In this talk a paper construction of this equidecomposition will be displayed.


Figure 5. Map to recover the cube.


Figure 6. Map to recover the parallelepiped.

 

References

Aguiló, F., Fiol, M. A., and Fiol, M. L. (2000) Periodic tilings as a dissection method, American Mathematical Monthly, 107, No. 4, 341-352.

Frederickson, G. N. (1997) Dissections: Plane & Fancy, Cambridge: Cambridge University Press, 310 pp.

* Work partially supported by the Comisión Interministerial de Ciencia y Tecnología, CICYT, under project TIC2000-1017 and by the Catalan Research Council under project.
 
 

NEXT

HOME