Cantorian Fractal Space-Time
Fluctuations in Turbulent Fluid Flows and Kinetic Theory of Gases
A. M. Selvam
Deputy Director (Retired)
Indian Institute of Tropical
Meteorology, Pune 411 008, India
Fluid flows such as gases
or liquids exhibit space-time fluctuations on all scales extending down
to molecular scales. Such broadband continuum fluctuations characterise
all dynamical systems in nature and are identified as selfsimilar fractals
in the newly emerging multidisciplinary science of nonlinear dynamics
and chaos. A cell dynamical system model has been developed by the
author to quantify the fractal space-time fluctuations of atmospheric
flows. The earth's atmosphere consists of a mixture of gases and obeys
the gas laws as formulated in the kinetic theory of gases developed
on probabilistic assumptions in 1859 by the physicist James Clerk Maxwell.
An alternative theory using the concept of fractals and chaos
is applied in this paper to derive the fundamental equation of the kinetic
theory of ideal gases and the Maxwell’s distribution of molecular
speeds.
Keywords: fractals,
chaos, kinetic theory of gases, gas laws
1.Introduction
Kinetic theory of ideal
gases is a study of systems consisting of a great number of molecules,
which are considered as bodies having a small size and mass (Kikoin and
Kikoin, 1978). Classical statistical methods of investigation (Dennery,
1972; Yavorsky and Detlaf, 1975; Kikoin and Kikoin, 1978; Rosser, 1985;
Guenault, 1988; Gupta, 1990; Ruhla, 1992; Dorlas, 1999; Chandrasekhar,
2000) are employed to estimate average values of quantities characterizing
aggregate molecular motion such as mean velocity, mean energy etc., which
determine the macro-scale characteristics of gases. The mean properties
of ideal gases are calculated with the following assumptions. (1) The intra-molecular
forces are completely absent instead of being small. (2) The dimensions
of molecules are ignored, and considered as material points. (3) The above
assumptions imply the molecules are completely free, move rectilinearly
and uniformly as if no forces act on them. (4) The ceaseless chaotic movements
of individual molecules obey Newton’s laws of motion.
The observed nonlinear space-time fluctuations of microscopic objects such
as atoms and molecules in an ideal gas are now (since 1980s) identified
as fractals generic to macro-scale real world dynamical systems
in nature such as, fluid flows, stock market price fluctuations, heart
beat patterns etc. The apparently chaotic (nonlinear) fractal fluctuations
however exhibit self-similarity, i.e., long-range space-time correlations.
The identification of the physical laws governing fractal fluctuations
is an intensive field of research in the newly (since 1980s) emerging science
of Nonlinear Dynamics and Chaos (Gleick, 1987). Mary Selvam (1990)
has developed a general systems theory for the simulation and prediction
of the observed fractal space-time fluctuations in dynamical systems
of all size scales ranging from the microscopic scale of atoms and molecules
to macro-scale turbulent fluid flows. The model concepts are applied to
derive the following classical relationships for an ideal gas: (1) pressure
exerted by an ideal gas (2) the Boltzmann distribution for molecular
energies (3) the Maxwell distribution of molecular velocities. The
derivation of the above relationships according to classical statistical
methods is briefly described followed by a detailed discussion of the fractal
concepts applied to derive the same equations.
The important new contributions of the general systems theory applied to
model ideal gases are as follows: (1) fractal fluctuations are signatures
of quantum-like chaos on all scales ranging from subatomic dynamics of
quantum systems to real world macro-scale fluid flows (2) quantum mechanical
laws are applicable to dynamical systems of all size scales.
The general systems theory concepts used in the derivation of the fundamental
equations for the kinetic theory of gases have been applied earlier by
the author for the simulation and prediction of both microscopic and macro-scale
dynamical systems (Selvam, 1990; Selvam, 1993; Selvam, and Fadnavis, 1998;
Selvam and Suvarna Fadnavis, 1999a; Selvam, and Suvarna Fadnavis, 1999b;
Selvam, 2001).
In the following, Sections 2, 3 and 4 deal respectively with application
of the model concepts to derive the following three classical relationships
for an ideal gas: (1) pressure exerted by an ideal gas (2) the Boltzmann
distribution for molecular energies (3) the Maxwell distribution
of molecular velocities. The derivation of the above relationships according
to classical statistical methods is briefly described followed by a detailed
discussion of the fractal concepts applied to derive the same equations.
In conclusion Section 5 discusses the universal characteristics of fractal
space-time fluctuations, a signature of quantum-like chaos exhibited by
dynamical systems of all size scales ranging from sub-atomic dynamics of
quantum systems to macro-scale turbulent fluid flows. The model shows that
quantum mechanical laws are applicable to macro-scale real world dynamical
systems and also provides physically consistent interpretations for wave-particle
duality and non-local connection exhibited by microscopic-scale quantum
systems which so far do not have a satisfactory explanation on the basis
of current concepts in quantum mechanics.
2. Pressure exerted by
an ideal gas
2.1 Classical statistical
physics
A brief summary of the
method for calculating pressure based on classical statistical physics
concepts is given in the following The molecular collisions exert a force
on the walls of the vessel containing the gas and this force is measured
by the parameter pressure, which is equal to the force per unit
area perpendicular to the direction of the force.
The pressure
p is calculated as
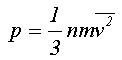where n is the
number of molecules per unit volume, m is the mass of one molecule
and 
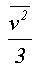represents the mean square velocity in any one direction
x, y
or z. The pressure p may be written as
(1)
In Equation (1) Ek
is equal to the mean kinetic energy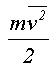 
of one molecule of a gas. Consequently the pressure of a gas equals two-thirds
of the mean kinetic energy of the molecules contained in a unit of its
volume. This is one of the most important conclusions of the kinetic theory
of an ideal gas. Equation (1) establishes a relationship between molecular
quantities, i.e., quantities relating to a separate molecule, and the value
of the pressure characterizing a gas as a whole – a macroscopic quantity
directly measured in experiments. Equation (1) is sometimes called the
fundamental
equation of the kinetic theory of ideal gases.
2.2 General systems theory
One of the most convincing
demonstrations that gases really are made up of fast moving molecules is
Brownian
motion, the observed constant jiggling around of tiny particles, such as
fragments of ash in smoke. This motion was first noticed in 1827 by the
British botanist, Robert Brown who initially assumed he was looking at
living creatures, but then found the same motion in what he knew to be
particles of inorganic material. Einstein showed how to use Brownian motion
to estimate the size of atoms (Kikoin and Kikoin, 1978; Fowler, 1997; Lee
and Kelvin).
Chaotic fluctuations such as those executed by molecules in a gas are now
identified as fractals generic to space-time fluctuations of dynamical
systems in nature (Mandelbrot, 1977; 1983; Gaspard et al., 1998).
Identification of the physics of fractal fluctuations and quantification
is an intensive field of research in the newly emerging (since 1980s) multidisciplinary
science of Nonlinear Dynamics and Chaos (Gleick, 1987). It has been
long supposed that the existence of chaotic behaviour in the microscopic
motions of atoms and molecules in fluids or solids is responsible for their
equilibrium and non-equilibrium properties. Recently this hypothesis of
microscopic chaos has been verified experimentally by Gaspard et al.
(1998) who found evidence for microscopic chaos in fluid systems, by the
observation of Brownian motion of a colloidal particle suspended in water.
Mary Selvam (1990) has developed a general systems theory (Capra, 1996)
for the observed space-time fractal fluctuations in dynamical systems,
which enable quantification of large-scale fluctuations in terms of inherent
smaller scale fluctuation characteristics. The irregular fractal
fluctuations occur on all space-time scales and may be considered to result
from the superimposition of a continuum of eddies or waves such as sine
waves. An eddy is basically a circular motion characterized by the radius
r
and r.m.s (root mean square) circulation speed w*.
Larger scale fluctuations result from the integration of enclosed smaller
scale fluctuations. The relationship between the r.m.s circulation speeds
W
and w*
of large and small eddy of respective radii R and r is given
as (Townsend, 1956; Mary Selvam, 1990)
(2)
The above equation represents
the growth of an eddy continuum with formation of a hierarchy of successively
larger eddies from enclosed smaller scale eddies. The square of the eddy
amplitude, i.e., W2
represents the eddy energy (kinetic). The large eddy energy is the integrated
mean of the enclosed smaller scale eddy energies and therefore the eddy
energy spectrum will follow statistical normal distribution according to
the Central Limit Theorem (Ruhla, 1992). Such a result that the
additive amplitudes (W) of eddies, when squared (W2)
represent the statistical normal distribution is exhibited by subatomic
dynamics of quantum systems such as the electron or photon (Maddox, 1998;
1993).
By analogy with quantum mechanics the square of the eddy amplitude W2
represents the kinetic energy E given as (from Equation 2)
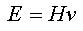In the above Equation
the parameter n
(proportional to 1/R) is the frequency of the large eddy and H
is a constant equal to 
for the growth of large eddies sustained by constant energy input proportional
to w*2
from fixed primary small scale eddy fluctuations. Energy content of eddies
is therefore similar to quantum systems which can possess only discrete
quanta or packets of energy content hn
where h is a universal constant of nature (Planck's constant) and
n
is the frequency in cycles per second of the electromagnetic radiation.
The macro-scale eddy continuum represented by Equation (2) obeys quantum-like
mechanical laws, a manifestation of quantum-like chaos. The apparent paradox
of wave-particle duality exhibited by microscopic scale quantum systems
such as an electron or photon is however physically consistent in the context
of real world macro-scale dynamical systems as explained in the following.
The bi-directional energy flow intrinsic to eddy circulations is associated
with bimodal, i.e., formation and dissipation respectively of phenomenological
form for manifestation of energy such as the formation of clouds in updrafts
and dissipation of clouds in adjacent downdrafts resulting in the observed
discrete cellular geometry to cloud structure. The commonplace occurrence
of clouds in a row is a manifestation of wave-particle duality in macro-scale
atmospheric flows. By analogy, the molecules (atoms) of an ideal gas may
be visualised as the manifestation of matter during a half-cycle of an
eddy circulation (Mary Selvam, 1990; Selvam and Fadnavis, 1999a). The primary
perturbation of r.m.s circulation speed w*
and eddy radius r represents the wave-like structure of a molecule
or atom in the ideal gas, the manifestation of matter of molecular mass
m
occurring during half a cycle of the complete circulation as explained
above.
The length scale ratio
R/r
in the above Equation (2) represents the fractional volume intermittency
of occurrence of small-scale (fractal) structures (Mary Selvam,
1993) across unit area of large eddy surface as shown in the following.
Considering two large
eddy circulations of respective radii R1
and R2
(R2 being
greater than R1)
and corresponding r.m.s circulation speeds W1
and W2
which grow from the same small-scale primary perturbation of radius r
and r.m.s circulation speed w*,
we have from Equation (2)
(3)
Introducing the factor 
representing eddy volumes on both sides of the above equation we have
(4)
Therefore
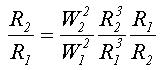
(5)
Substituting for R1/
R2 on the right
hand side from Equation (3) we have the following relation for fractional
volume intermittency of occurrence of small-scale fluctuations given by
the fourth moment about the mean for the relative eddy transports as
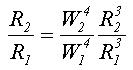
(6)
The length scale ratio
R1/
R2 is equal to
the transport of fractional volume of small-scale fluctuations in the environment
of the large eddy (per unit volume of large eddy), basically by eddy mixing
process. Considering large and small eddies of respective radii R
and r and r.m.s circulation speeds W and w*
the corresponding mass transport M of gas across unit area for half
cycle of large eddy circulation in terms of molecular mass is equal to 
. The molecular mass m corresponds to the small-scale primary eddy
perturbation. Multiplying both sides of Equation (2) by nm/2 and
rewriting
(7)
In
the above equation the large eddy circulation speed W represents
the acceleration since it is computed as an incremental value relative
to its earlier stage of eddy growth. The pressure p exerted by the
gas is given by the product MW equal to the rate of change of momentum
due to molecular impact across unit area of the large eddy surface. Equation
(7) may now be written as
(8)
The r.m.s eddy circulation
speed w* represents
by concept the average molecular speed in any direction and the average
kinetic energy of one molecule designated by Ek
is equal to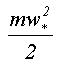 
. The above Equation (8) may now be written as
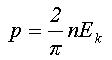
(9)
Equation (9) is almost
the same as Equation (1), the fundamental equation of the kinetic theory
of ideal gases, namely, 
.
The important differences
in the physical concepts underlying the derivation of the fundamental
equation of the kinetic theory of ideal gases by classical statistical
physical methods and the general systems theory for fractal space-time
fluctuations are as follows: (1) The general systems theory visualises
molecules or atoms as manifestation of matter during half a cycle of eddy
circulation. Classical physics visualises molecules and atoms as point
objects. (2) The r.m.s velocity w*
represents the average velocity for computation of mean molecular kinetic
energy in the general systems theory. The mean square velocity of the molecule
or atom in any one direction (x, y or z) equal to 
is used for computing the molecular kinetic energy in classical physics.
3. Boltzmann distribution
for molecular energies in an ideal gas
3.1 Classical physics
For any system large or
small in thermal equilibrium at temperature T, the probability P
of being in a particular state at energy E is proportional to 
where kB is the Boltzmann’s
constant. This is called the Boltzmann distribution and may be written
as
(10)
3.2 General systems theory
The physical concepts
of the general systems theory enables to show that precise ordered mathematical
patterns underlie the apparently chaotic space-time fluctuations of dynamical
systems. The irregular fractal fluctuations of dynamical systems
may be visualized to result from the superimposition of an ensemble of
eddies, namely an eddy continuum. An eddy continuum by concept consists
of a hierarchy of eddies, the larger scale eddies enclosing smaller scale
eddies. The larger scale eddies grow by the spatial integration of enclosed
smaller scale eddies and the growth trajectory traces an overall logarithmic
spiral flow path with the quasiperiodic Penrose tiling pattern for
the internal structure (Mary Selvam, 1990; Selvam and Fadnavis, 1998).
The ratio of radii (R2/
R1) or r.m.s.
circulation speeds (W2/W1)
corresponding to the successive growth steps of the large eddy generating
the geometry of the quasiperiodic Penrose tiling pattern is equal
to the golden mean t
(@
1.618).
(11)
The r.m.s circulation
speed W of the large eddy follows a logarithmic relationship with
respect to the length scale ratio z equal to R/r as given
below
(12)
In Equation (12) the variable
k
represents for each step of eddy growth, the fractional volume dilution
of large eddy by turbulent eddy fluctuations carried on the large eddy
envelope and is given as
(13)
Incidentally, Equation
(12) represents the observed logarithmic spiral air flow structure in the
planetary atmospheric boundary layer and the constant k called the
von
Karman’s constant is determined from observations to be equal to about
0.38
(Mary Selvam, 1990; Selvam and Fadnavis, 1998).
From Equations (11)
and (13) it is seen that, for successive large eddy growth steps generating
the quasiperiodic Penrose tiling pattern, the value of k
is equal to 1/t2
(@0.38)
where t
is the golden mean (@1.618).
Substituting for k in Equation (12) we have
(14)
Therefore
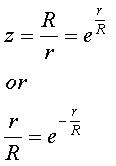
(15)
The ratio r/R represents
the fractional probability P of occurrence of small-scale fluctuations
(r) in the large eddy (R) environment. Considering two large
eddies of radii R1
and R2
(R2
greater than R1)
and corresponding r.m.s circulation speeds W1
and W2
which grow from the same primary small-scale eddy of radius r and
r.m.s circulation speed w*
we have from Equation (2)
(16)
From Equations (15) and
(16)
(17)
The square of r.m.s circulation
speed W2
represents eddy kinetic energy. Following classical physical concepts (Kikoin
and Kikoin, 1978) the primary (small-scale) eddy energy may be written
in terms of the eddy environment temperature T and the Boltzmann’s
constant kB as
(18)
Representing the larger
scale eddy energy as E
(19)
The length scale ratio
R1/R2
therefore represents fractional probability P of occurrence of large
eddy energy E in the environment of the primary small-scale eddy
energy kBT
(Equation 18). The expression for P is obtained from Equation (17)
as
(20)
The above Equation (20)
is the same as the Boltzmann’s equation (Equation 10).
The derivation of
Boltzmann’s
equation from general systems theory concepts visualises the eddy energy
distribution as follows: (1) The primary small-scale eddy represents the
molecules whose eddy kinetic energy is equal to kBT
as in classical physics. (2) The energy pumping from the primary small-scale
eddy generates growth of progressive larger eddies (Mary Selvam, 1990).
The r.m.s circulation speeds W of larger eddies are smaller than
that of the primary small-scale eddy (Equation 2). (3) The space-time fractal
fluctuations of molecules (atoms) in an ideal gas may be visualised to
result from an eddy continuum with the eddy energy E per unit volume
relative to primary molecular kinetic energy (kBT)
decreasing progressively with increase in eddy size.
4. Maxwell-Boltzmann distribution
of molecular speeds
4.1 classical physics
The distribution of molecular
speeds was derived by Maxwell based on the probabilistic assumptions,
namely (i) uniform distribution in space, (ii) mutual independence of the
three velocity components and (iii) isotropy as regards the directions
of the velocities (Ruhla, 1992). These assumptions were also used in deriving
the fundamental gas law at Equation (1) for a perfect gas. Maxwell's
distribution of molecular speeds is given by the following equation.
(21)
where r(v)
is the probability density assigned to the speed v, T is
the absolute temperature of the perfect gas, m is the mass of a
molecule and kB
is the Boltzmann's constant. For a given gas at a fixed temperature
T,
the probability density r(v)
may be written as
(22)
A graph of Maxwell's
distribution of molecular speeds is shown in Figure 1.
4.2 General systems theory
The steady state upward
transport of small-scale fluctuation of speed w*
and size scale r in the environment of larger scale fluctuation
of speed W and size R is given as (Mary Selvam, 1990; Selvam
and Fadnavis, 1998)
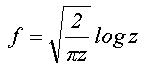
(23)
In Equation (23) z
is the length scale ratio equal to R/r. Considering three-dimensional
fluctuations the fractional contribution (probability density) of smaller
length scale r fluctuations in the environment of the larger length
scale R fluctuation is given by f 3.
The eddy circulation speeds follow the logarithmic law with respect to
the length scale ratio z (Equation 12), namely
The eddy circulation speeds
are therefore proportional to log z, that is
(24)
A graph of f 3
versus log z will give the probability density distribution for
molecular speeds. The cell dynamical system model predicted molecular speed
distribution in a perfect gas is shown as crosses in Figure 1. The distributions
(Maxwell's and model predicted) are normalized with respect to the
maximum speed. There is close agreement between the Maxwell's and
model-predicted distributions for molecular speeds in a perfect gas.
Figure 1
5. Conclusions
Dynamical systems of all
size scales ranging from microscopic scale quantum systems to macro-scale
turbulent fluid flows exhibit self-similar fractal space-time fluctuations.
Self-similarity implies long-range space-time correlations or non-local
connections such as that observed in quantum systems. A general systems
theory developed by the author enables to show quantitatively that the
observed fractal space-time fluctuations generic to dynamical systems
in nature are signatures of quantum-like chaos. The model concepts for
Cantorian
fractal space-time fluctuations is applied to derive the fundamental
gas law, namely 
and also the Maxwell’s molecular speed distribution for a perfect
gas. The model predictions are in agreement with Maxwell's kinetic
theory of gases developed in 1859 on probabilistic assumptions.
Acknowledgements
The author is grateful
to Dr. A. S. R. Murty for his keen interest and encouragement during the
course of the study.
References
Bak, P., C. Tang, K. Wiesenfeld,
1987: Self-organized criticality: an explanation of 1/f noise. Phys.
Rev. Lett. 59, 381-384.
Bak, P., C. Tang, K. Wiesenfeld,
1988: Self-organized criticality. Phys. Rev. A. 38, 364-374.
Chandrasekhar, B. S.,
2000: Why are Things the Way They are, Cambridge University Press,
Cambridge, pp.251.
Capra, F., 1996: The
Web of Life, Harper Collins, London, pp. 311.
Dennery, P., 1972: An
Introduction to Statistical Mechanics, George Allen and Unwin Ltd.,
London, pp.116.
Dorlas, T. C., 1999: Statistical
Mechanics, Institute of Physics Publishing, Bristol, London, pp.267.
Fowler, M., 1997: Brownian
motion, http://www.phys.virginia.edu/classes/252
Gaspard, P., M. E. Briggs,
M. K. Francis, J. V. Sengers, R. W. Gammon, J. R. Dorfman and R. V. Calabrese,
1998: Experimental evidence for microscopic chaos, Nature 394,
865-867.
Gupta, M. C., 1990: Statistical
Thermodynamics, Wiley Eastern Ltd., New Delhi, pp.401.
Gleick, J., 1987: Chaos:
Making a New Science. Viking , New York.
Kikoin, A. K. and I. K.
Kikoin, 1978: Molecular Physics, Mir Publishers, Moscow, pp.471.
Lee, Y. K. and H. Kelvin,
---Brownian Motion-The Research Goes On http://www.doc.ic.ac.uk/~nd/surprise_95/journal/vol4/ykl/report.html
Maddox, J., 1988: Licence
to slang Copenhagen? Nature 332, 581.
Maddox, J., 1993: Can
quantum theory be understood? Nature 361, 493.
Mandelbrot, B.B., 1977:
Fractals:
Form, Chance and Dimension, Freeman, San Francisco.
Mandelbrot, B.B., 1983:
The
Fractal Geometry of Nature, W.H. Freeman, pp.468.
Newman, M., 2000: The
power of design, Nature 405, 412-413.
Rosser, W. G., 1985: An
Introduction to Statistical Physics, Ellis Horwood Publishers, Chichester,
West Sussex, England, pp. 377.
Ruhla, C., 1992: The
Physics of Chance, Oxford university press, pp. 217.
Selvam, A. M., 1990: Deterministic
chaos, fractals and quantum-like mechanics in atmospheric flows, Canadian
J. Physics 68, 831-841. http://xxx.lanl.gov/html/physics/0010046
Selvam, A. M., 1993: Universal
quantification for deterministic chaos in dynamical systems, Applied
Mathematical Modelling 17, 642-649. http://xxx.lanl.gov/html/physics/0008010
Selvam, A. M. and S. Fadnavis,
1998: Signatures of a universal spectrum for atmospheric interannual variability
in some disparate climatic regimes, Meteorology and Atmospheric Physics
66, 87-112, (Springer-Verlag, Austria) http://xxx.lanl.gov/abs/chao-dyn/9805028.
Selvam, A. M. and Suvarna
Fadnavis, 1999a: Superstrings, Cantorian-fractal space-time and quantum-like
chaos in atmospheric flows, Chaos, Solitons and Fractals 10(8),
1321-1334. http://xxx.lanl.gov/abs/chao-dyn/9806002.
Selvam, A. M., and Suvarna
Fadnavis, 1999b: Cantorian fractal spacetime, quantum-like chaos and scale
relativity in atmospheric flows, Chaos, Solitons and Fractals 10(9),
1577-1582. http://xxx.lanl.gov/abs/chao-dyn/9808015.
Selvam, A. M., 2001: Quantum-like
chaos in prime number distribution and in turbulent fluid flows. http://xxx.lanl.gov/html/physics/0005067
Published with modification in the Canadian electronic journal APEIRON
8(3), 29-64, (2001). http://redshift.vif.com/JournalFiles/V08NO3PDF/V08N3SEL.PDF
Yavorsky, B. and A. Detlaf,
1975: Handbook of Physics, Mir Publishers, Moscow, pp.965.