- In order to precisely approximate the helium ground-state energy, we cannot rely on
a simple model (e.g. separation of variables) alone [Lev2, p.258, l.-13].
The trial functions should have the form [Lev2, p.258, (9.68)] that can simulate
the features of reality.
- To be precise, when the transmission coefficient T has a local minimum at 2k'a=(2n+1)p/2, one should not say the local minimum is near 2k'a=(2n+1)p/2 [Mer2, p.109, l.5]. Otherwise, it will appear that the author is attempting to concede precision in the hope that the minimum will fall in the neighborhood of 2k'a=(2n+1)p/2. Thus, the reader will have the impression that the author is unable to prove the precise statement: T has a local minimum at 2k'a=(2n+1)p/2.
Proof.
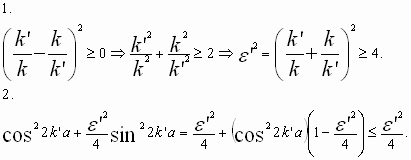
- Using corrections to identify the mistakes.
If there is a discrepancy between the theoretical value and the experimental value, then we must have committed some mistakes. For example, according to classical statistical mechanics, the electronic contribution to the heat capacity should be (3/2)NkB [Kit2, p.151, l.14]. However, the observed heat capacity is smaller than (3/2)NkB by a factor of order 0.01 or less [Kit2, p.151, l.16]. Fermi uses the Pauli exclusion principle to make certain corrections. Using his correction, the theoretical value and observed values agree. Thus, we successfully identify the shortcoming of the previous theory.
- When proving a formula or a theorem, we must carefully justify our argument
as we proceed. In [Kit, p.93, l.-4-l.-2], Kittel fails to explain why we can replace the sum by an integral, while [Iba, p.107, l.-1-p.108, l.2] identifies the reason precisely.