Hyperbolic Surfaces in Nature
A table-top is an example of an ordinary Euclidean or flat space.
Like any two-dimensional flat surface, it has zero curvature. What about
non-zero curvature? There are two kinds (naturally): positive and
negative. A familiar example of a positively curved surface is a
sphere. But what about negatively curved surfaces? Are there any
familiar examples of those? One is the saddle of a horse.
 If you start at the front end of a saddle and move backwards, the saddle
curves down and then up. If you move from side to side, however,
it curves up and then down. This is the definition of negative
curvature -- opposite curvature going in different directions. On a
sphere, no matter which way you go, it curves the same way, so we call
it positive curvature.
If you start at the front end of a saddle and move backwards, the saddle
curves down and then up. If you move from side to side, however,
it curves up and then down. This is the definition of negative
curvature -- opposite curvature going in different directions. On a
sphere, no matter which way you go, it curves the same way, so we call
it positive curvature.
Another example you might have seen is this seedpod from a particular
kind of tree, which has many saddle-shaped regions instead of just
one:

Make your own Hyperbolic Surface
You can make a hyperbolic surface yourself. The key to doing this is a
remarkable geometric fact about surfaces and curvature. On a flat plane,
the three angles of a triangle add up to 180 degrees. On a sphere, the
angles add up to more than 180, and on a hyperbolic surface, they
add up to less. In a sense, there's an excess of area around a
point on a hyperbolic surface. Spheres, on the other hand, have the minimum
surface area for a given volume. That's why bubbles are spherical.
Hyperbolic space, which is three-dimensional, has more volume than ordinary
Euclidean space! (Just as a hyperbolic surface has more area.) For more
technical details, check out this link.

Tiling the Plane
So, to make a hyperbolic plane surface, we need to arrange for there to be,
in some sense, more surface around a point than usual. We do this by
tiling the plane. You can tile a flat plane with hexagons -- this is
the pattern of the honeycomb in a beehive. But what if you try to
tile a plane with heptagons (seven sided instead of six)? You can't
tile a flat plane this way, but you can tile a hyperbolic plane
with heptagons (or octagons, etc.).
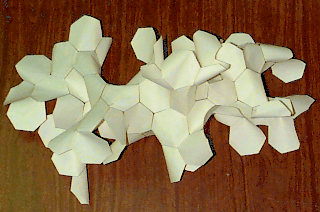
First we need to make some heptagons. Print out this
Postscript File to make a page of heptagons,
each one inch on a side. Print it out several times, and cut out
all the heptagons. Then start constructing your surface by taping the
edges of the heptagons together. Three heptagons come together at
each vertex, like in the honeycomb, only the angle is more than 120
degrees, so your paper model won't be flat. The hyperbolic surface
curves up and around like the seedpod above, until you have a model
that looks something like this:
Links
The Geometry Center at the
University of Minnesota has a number of exhibits devoted to Hyperbolic
Geometry, including a
Java Applet for
drawing Hyperbolic Triangles and some pictures from a
Computer
Generated Fly-through of Hyperbolic Space.

 If you start at the front end of a saddle and move backwards, the saddle
curves down and then up. If you move from side to side, however,
it curves up and then down. This is the definition of negative
curvature -- opposite curvature going in different directions. On a
sphere, no matter which way you go, it curves the same way, so we call
it positive curvature.
If you start at the front end of a saddle and move backwards, the saddle
curves down and then up. If you move from side to side, however,
it curves up and then down. This is the definition of negative
curvature -- opposite curvature going in different directions. On a
sphere, no matter which way you go, it curves the same way, so we call
it positive curvature.

