Momentum Conservation in Two Dimensions
NAME ____________________________ PARTNER ________________________ DATE _______
Is Momentum Conserved in Two Dimensions? Conservation of momentum can be used to solve a variety of collision and explosion problems. So far we have only considered momentum conservation in one dimension, but real collisions lead to motions in two and three dimensions. For example, air molecules are continually colliding in space and bouncing off in different directions.
Since momentum is a vector, the Law of Conservation of Momentum in two dimensions requires that if the vector conservation equation is broken into components then the conservation law must also hold for each of the vector components. Thus, if we consider the interaction of three or more objects, and if
![]()
then
![]()
and
![]()
If a coordinate system is chosen and a given momentum vector makes an angle q with respect to the designated x-axis then the momentum vector can be broken into components in the usual way:
![]()
Collisions in 2 Dimensions
OBJECTIVE
To illustrate conservation of momentum in two dimensions by the
Standard PSSC model and then repeating the procedure for the use of a Traditional Japanese Toy called the "Ohajiki"
MATERIALS
For this experiment you will need the following equipment:
PSSC type collision in 2D apparatus, 3 balls, 6-8 Ohajiki pcs., carbon paper, and string.
PROCEDURE
1. Set up the apparatus and tape four sheets of carbon paper together to form a square. Do the same with four sheets of paper. Put the carbon paper on top of the paper on the floor at the base of the apparatus so the plumb bob hangs over the center of the short edge. Put some weights or tape on the paper to hold it in place. Mark the position of the plumb bob (directly below the setscrew) on the paper. This is nearly your origin in x-y momentum space.
- Put one steel ball on the setscrew and adjust the screw so that the steel ball is the same height as a second ball on the edge of the ramp. The experiment will only illustrate your inability to follow instructions if you do not adjust the heights correctly!
- Remove the ball from the setscrew and release the steel ball from a point near the top of the ramp so it lands on the paper. Repeat this procedure from the same release point about ten times and circle the distribution. (the scatter indicates how consistent you are) Using what you know about projectile motion determine the horizontal speed of the ball as it leaves the ramp. Show your calculations.
4. Remove the carbon paper. With the second steel ball balanced on the setscrew so the balls collide at 0.0o, try several collisions, releasing the ball from the ramp exactly as in procedure 3. Did the target land nearly in the same place as in part 2? If it did, then nearly all the Kinetic Energy is transferred to the target. If it went off to one side your don't have it aligned for 0.0o. If it landed short or long then you did not align the heights correctly - go back to part 2 and align them properly. Keep adjusting until you are sure everything is aligned; then use the carbon paper.
5. Turn the support arm that holds the target ball to change the angle to around 20-30 degrees and try another collision. Did both balls clear the support? If they did you should hear one click not two clicks when the balls hit the floor? If you hear two clicks - go back to part 2 and align properly. Keep adjusting until you are sure everything is aligned; then use the carbon paper. Run several trials then circle the positions of the target marks and label T1.
6. Circle the positions of the marks on the paper and label them I2 and T2 for incident and target.
7. Turn the support arm that holds the target ball to another angle. The initial position (at the time of collision) of the target ball is marked on the paper by the plumb bob. Geometry and the radius of the balls must carefully determine the position of the incident ball.
8. Run several trials each at different angles. Mark the trials I3, T3, I4, T4 etc.
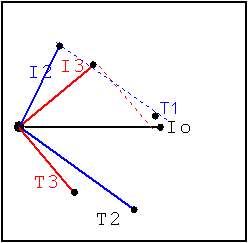
9. Carefully draw vectors representing the velocities of the incident and target for each trial. Use a different color for each collision. Then add the two vectors graphically, show the addition with a dotted line.
10. Run one trial for two balls of different masses. Choose the target so that the incident momentum remains the same.
11. Repeat activity with the following adaptations: Set up for "Ohajiki" as you would for a game of marbles and tape four sheets of paper together to form a square. Put some weights or tape on the paper to hold it in place. Mark the position of the incident "Ohajiki" or also called the "shooter" on the paper. This is nearly your origin in x-y momentum space.
12. Put one "Ohajiki" or "shooter" on the point of origin and adjust the second "Ohajiki" or "target" so that they are along the same line of sight. The experiment will only illustrate your inability to follow instructions if you do not adjust the positions correctly!
13. Shoot the "Ohajiki" from the point of origin and trace its motion from that point to the target Ohajiki so the collision is traced on the paper. Repeat this procedure from the same release point about ten times and circle the distribution. (the scatter indicates how consistent you are / try to maintain a constant release of your shooter) Using what you know about projectile motion and the law of conservation of momentum determine the horizontal speed of the ball as it leaves our finger. Show your calculations.
14. With the second shooter "Ohajiki" placed on the origin so the Ohajiki's collide at 0.0o, try several collisions, shooting the Incident Ohajiki from the origin exactly as in procedure 13. Did the target land nearly in the same place? If it did, then nearly all the Kinetic Energy is transferred to the target. If it went off to one side you don't have it aligned for 0.0o. If it landed short or long then you did not align the heights correctly - go back and align them properly. Keep adjusting until you are sure everything is aligned; then repeat.
15. Change the position of the shooter Ohajiki with respect to the target ball so as to change the angle between them around 20-30 degrees and try another collision. Did both balls continue along their path? Run several trials then circle the positions of the target marks and label T1.
16. Circle the positions of the target and shooter Ohajiki's on the paper and label them I2 and T2 for incident and target.
17. Change the position of the target ball to another angle. The initial position (at the time of collision) of the target ball is marked on the paper as before. Geometry and the radius of the Ohajiki must carefully determine the position of the incident ball.
18. Run several trials each at different angles. Mark the trials I3, T3, I4, T4 etc.
ANALYSIS
Determine the height of the table and the time for the balls to fall to the floor from the initial landing position, Io. Also find the mass of each ball.
| Time | Mass 1 | Mass 2 | Mass Glass |
Determine the scale that converts the velocity vectors drawn to momentum vectors for the steel balls, i.e. each centimeter of length represents _____________ kg m/s of momentum. Hint: it is related to the mass and time of fall.
Determine the scale that converts the velocity vectors drawn to momentum vectors for the glass ball, i.e. each centimeter of length represents _____________ kg m/s of momentum.
Measure the momentum vector for the incident ball and fill in the scaled vale in the chart below.
Measure the momentum vectors for the two balls after each collision; also measure the total vector sum of the two momenta. Fill in the scaled value in the chart below. For each case compare the vector sum of the momenta of the balls with the momentum of the incident ball. If the sum of the momenta does not lie within the uncertainty of your initial momentum (enter zero if it does lie within the uncertainty), then fill in the change in length and angle in the chart below.
| Trial | Initial Momentum | Incidents final Momentum | Targets final Momentum |
| 1 | |||
| 2 | |||
| 3 | |||
| 4 |
| Trial | Magnitude of total Momentum | Change of Momentum | Change in Angle of Momentum |
| 1 | |||
| 2 | |||
| 3 | |||
| 4 |
Does it appear that momentum is conserved within the uncertainties of the experiment? Explain.
Determine if the collisions are elastic when the balls are equal mass (after you watch the video on momentum you can prove the angle should be 90 degrees for and elastic collision - that is when the KE remains the same)?
Repeat all analysis for the Traditional Japanese Toy the "Ohajiki".